Understanding Roman Numerals that Multiply to 35

Introduction to Roman Numerals

Roman numerals originated in ancient Rome and were widely used throughout the Roman Empire for numbering and record-keeping purposes. Unlike the decimal system used today, which relies on combinations of the digits 0 through 9, Roman numerals are represented by combinations of letters from the Latin alphabet. Roman numerals, with their unique symbols and system of notation, have been used for centuries to represent numbers in various contexts. In this comprehensive guide, we’ll explore how Roman numerals can be multiplied to achieve the value of 35, providing insight into the intricacies of this ancient numerical system.

Basic Principles of Roman Numerals

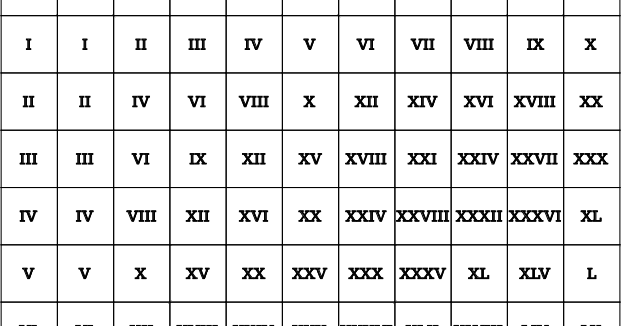
In Roman numerals, certain letters represent specific numerical values. The basic symbols and their corresponding values are as follows:

I = 1

V = 5

X = 10

L = 50

C = 100

D = 500

M = 1000

By combining these symbols, larger numbers can be represented. However, there are rules governing the formation of Roman numerals, including limitations on consecutive repetition of certain symbols and specific order of symbols for proper representation.

Multiplying Roman Numerals

To multiply Roman numerals, one must understand their numerical values and the principles of multiplication. Since Roman numerals lack a zero and do not represent fractions or decimals, multiplication can be achieved through repeated addition or the use of specific mathematical rules.

Determining Roman Numerals That Multiply to 35

To find Roman numerals that multiply by 35, we can explore different combinations of numerals and their values. Since 35 is not a typical product of two Roman numerals, we need to consider alternative approaches to achieve this value.

Example Calculations

Let’s examine some example calculations to illustrate how Roman numerals can be multiplied to obtain 35:

Using the numeral “V” (5) and “VII” (7) together: V * VII = 5 * 7 = 35

Another approach involves using the numeral “XXXV” directly: XXXV = 35

These examples demonstrate how different combinations of Roman numerals can yield the desired product of 35.

Roman Numeral Multiplication Techniques

While Roman numerals were primarily used for counting and basic arithmetic operations in ancient times, modern approaches to multiplication involve converting Roman numerals to Arabic numerals (decimal system), performing the multiplication, and then converting the result back to Roman numerals.

Alternatively, some online tools and calculators are available that can perform Roman numeral multiplication efficiently, allowing users to input the desired numbers and obtain the result without manual calculation.

Historical Context

Roman numerals were developed by the ancient Romans and were widely used throughout the Roman Empire for various purposes, including commerce, record-keeping, and monument inscriptions.

The Roman numeral system evolved, with changes in notation and usage occurring across different periods of history. Understanding the historical context can provide insights into the development and significance of Roman numerals.

Mathematical Properties of Roman Numerals

Roman numerals are based on an additive system, where numbers are represented by adding or subtracting symbols. Multiplication in Roman numerals involves repeated addition, making it a less intuitive operation compared to the decimal system.

Roman numerals do not include a zero, which can complicate arithmetic operations such as multiplication and division. The absence of a zero also limits the range of numbers that can be represented in Roman numerals.

Practical Applications

While Roman numerals are no longer commonly used for mathematical calculations in modern society, they still hold cultural and practical significance in various contexts. For example, they are often used in the numbering of chapters, volumes, and sections in books and legal documents.

Roman numerals are also frequently employed in clock faces, numbering of movie sequels, and the designation of centuries in historical and artistic contexts.

Educational Resources

For individuals interested in learning more about Roman numerals and their mathematical properties, there are numerous educational resources available, including books, online courses, and interactive tutorials.

Many educational websites offer exercises and quizzes to help reinforce understanding of Roman numerals and their application in arithmetic operations.

FAQs

Q: Can any combination of Roman numerals be multiplied to achieve 35? A: No, not every combination of Roman numerals will result in a product of 35. Specific combinations must be chosen carefully to ensure the correct result.

Q: Are there alternative methods for representing 35 in Roman numerals? A: Yes, besides multiplication, 35 can also be represented directly as “XXXV,” which is the Roman numeral equivalent of 35.

Q: Why is 35 not a common product of two Roman numerals? A: The Roman numeral system is based on additive principles rather than multiplication, leading to fewer commonly encountered products of two numerals.

Q: How can I learn more about Roman numerals and their mathematical properties? A: There are various resources available, including books, online tutorials, and educational websites, that provide comprehensive explanations and examples of Roman numerals and their mathematical operations.

Conclusion

In conclusion, while Roman numerals may present some challenges when it comes to multiplication, it is possible to represent the value of 35 using specific combinations of numerals. By understanding the principles of Roman numerals and their corresponding values, one can explore different strategies for achieving desired numerical outcomes. Whether through direct representation or calculated multiplication, Roman numerals offer a fascinating glimpse into the history of mathematics and the ingenuity of ancient civilizations.